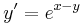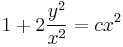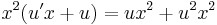Szerkesztő:Mozo/A3 gyakorló feladatok 5.

A MathWikiből
(Változatok közti eltérés)
(→Fokszámban homogén egyenletek)
(→Fokszámban homogén egyenletek)
47. sor: 47. sor:
 
:<math>u'x=u+u^2\,</math>
 
:<math>u'x=u+u^2\,</math>
 
:<math>\int\frac{\mathrm{d}u}{u(u+1)}=\int \frac{1}{x}\mathrm{d}x\,</math>
 
:<math>\int\frac{\mathrm{d}u}{u(u+1)}=\int \frac{1}{x}\mathrm{d}x\,</math>
−
:<math>\int\frac{1}{u}-\frac{u+1}\mathrm{d}u=\int \frac{1}{x}\mathrm{d}x\,</math>
+
:<math>\int\frac{1}{u}-\frac{1}{u+1}\mathrm{d}u=\int \frac{1}{x}\mathrm{d}x\,</math>
 
:<math>\ln|u|-\ln|u+1|=\ln |x|+C\,</math>; <math>(C\in\mathbf{R})</math>
 
:<math>\ln|u|-\ln|u+1|=\ln |x|+C\,</math>; <math>(C\in\mathbf{R})</math>
 
:<math>\ln\left|\frac{u}{u+1}\right|=\ln c_1|x|\,</math>; <math>(c_1=\ln C\,)</math>
 
:<math>\ln\left|\frac{u}{u+1}\right|=\ln c_1|x|\,</math>; <math>(c_1=\ln C\,)</math>
55. sor: 55. sor:
 
Explicit mo.:
 
Explicit mo.:
 
:<math>y=\frac{cx^2}{1+cx}\,</math>; <math>(c\in\mathbf{R})</math>
 
:<math>y=\frac{cx^2}{1+cx}\,</math>; <math>(c\in\mathbf{R})</math>
 +
===Kezdetiérték feladat===
 +
'''1.''' <math>y'=e^{x-y}\,</math>

A lap 2016. június 3., 22:12-kori változata

<Matematika A3a 2008

Differenciálegyenletek

Fokszámban homogén egyenletek

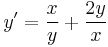
1. y'=\frac{x}{y}+\frac{2y}{x}

MO. u = y / x; y = ux; y' = u'x + u

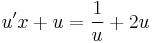
u'x+u=\frac{1}{u}+2u
u'x=\frac{1+2u^2}{u}
\int\frac{u}{1+2u^2}\,\mathrm{d}u=\int \frac{1}{x}\,\mathrm{d}x
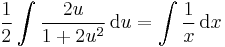
\frac{1}{2}\int\frac{2u}{1+2u^2}\,\mathrm{d}u=\int \frac{1}{x}\,\mathrm{d}x
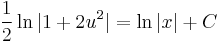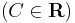
\frac{1}{2}\ln|1+2u^2|=\ln|x|+C; (C\in\mathbf{R})
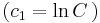
\ln |1+2u^2|=\ln c_1^2x^2\,; (c_1=\ln C\,)
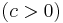
1+2u^2=cx^2\,; \qquad(c>0)\,

Implicit mo.:

1+2\frac{y^2}{x^2}=cx^2\,

Explicit mo.:

y=x\cdot\left(\pm\sqrt{\frac{c}{2}}\sqrt{x^2-\frac{1}{c}}\right)
Itt |x|>\frac{1}{\sqrt{c}}

2. x^2y'=xy+y^2\,

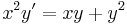
MO. y≡0 konstans mo. y=ux helyettesítéssel:

x^2(u'x+u)=ux^2+u^2x^2\,

ahonnan intervallumon értelmezett megoldás esetén:

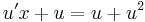
u'x+u=u+u^2\,
u'x=u^2\,
\int\frac{\mathrm{d}u}{u^2}=\int \frac{1}{x}\mathrm{d}x\,
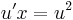
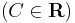
-\frac{1}{u}=\ln |x|+C\,; (C\in\mathbf{R})

Implicit mo.:

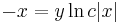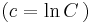-x=y\ln c|x|\,; (c=\ln C\,) és y=0

Explicit mo.:

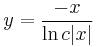y=\frac{-x}{\ln c|x|}\, és y=0.

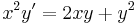
3. x^2y'=2xy+y^2\,

MO. y≡0 konstans mo. y=ux helyettesítéssel:

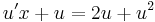
u'x+u=2u+u^2\,
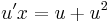
u'x=u+u^2\,
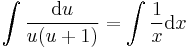
\int\frac{\mathrm{d}u}{u(u+1)}=\int \frac{1}{x}\mathrm{d}x\,
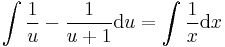
\int\frac{1}{u}-\frac{1}{u+1}\mathrm{d}u=\int \frac{1}{x}\mathrm{d}x\,
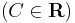
\ln|u|-\ln|u+1|=\ln |x|+C\,; (C\in\mathbf{R})
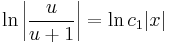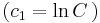
\ln\left|\frac{u}{u+1}\right|=\ln c_1|x|\,; (c_1=\ln C\,)
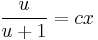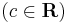
\frac{u}{u+1}= cx\,; (c\in\mathbf{R})

Implicit mo.:

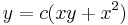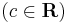
y= c(xy+x^2)\,; (c\in\mathbf{R})

Explicit mo.:

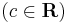
y=\frac{cx^2}{1+cx}\,; (c\in\mathbf{R})

Kezdetiérték feladat

1. y'=e^{x-y}\,

A lap eredeti címe: „http://wiki.math.bme.hu/index.php?title=Szerkeszt%C5%91:Mozo/A3_gyakorl%C3%B3_feladatok_5.&oldid=11730”
Személyes eszközök